Coordinate Systems and Aspects
Mark Pottenger
We had a visitor in early November (Maurice Silver from Australia) who brought the issue of aspect measurement to the foreground of our attention for a while.
Coordinate Systems
Coordinate systems are systems of numbering and measurement which allow us to describe or map the locations of objects in the sky (or anywhere) where things are, were or will be. The space-time system we are most familiar with involves four dimensions (measurements needed to completely describe a location): three dimensions of space and one of time. Any single chart is a frozen diagram of a particular moment in time, allowing us to describe locations within the chart with only the three spatial dimensions. We can simplify beyond that only by ignoring or losing information. Most horoscopes we look at have dropped two spatial dimensions, leaving us with only longitude. Astrologers deal with the two dropped coordinates to varying extents. Some look at latitude (or declination) and some look at distance.
I will limit this discussion to two-dimensional astrology, leaving the distance issue (how are aspects affected when one point is 40 (or 200,000) times farther away than another?) for another day.
Two-dimensional astrology involves the simplifying assumption that everything we see in the sky is at the same distance on the surface of a sphere (the celestial sphere). Positions on the surface of a sphere can be completely specified by two numbers. The usual convention is for one number to measure around a circle cutting through the center of the sphere and for the other number to measure at a perpendicular (at a 90 degree angle) to that circle. A circle through the center of a sphere is called a great circle because it is the largest possible circle that can be cut through that sphere. Any circle that doesn't cut through the center is smaller. An infinite number of great circles exist on any sphere since cutting through the center is the only requirement—no particular orientation is preferred.
A few great circles on the celestial sphere have names. The celestial equator, the ecliptic, the galactic equator, the horizon, the meridian and the prime vertical are the ones most used in astronomy and astrology. These all move on various time scales, but in any given chart they are frozen in a particular relationship to each other. Measurements using some of the great circles include:
|
GREAT CIRCLE |
ALONG |
UP/DOWN |
|
Ecliptic |
Longitude |
Latitude |
|
Equator |
Right Ascension |
Declination or Hour angle |
|
Horizon |
Azimuth |
Altitude |
|
Prime Vertical |
Longitude |
Latitude |
|
Galactic |
Longitude |
Latitude |
Each longitude or latitude is prefixed with the great circle name to indicate which one you mean, with the words celestial and zodiacal also going to the ecliptic.
The two numbers describing a location on a sphere are distance along the great circle defining the system of measurement from some starting point to a point at which a perpendicular line of 90 degrees or shorter length crosses the location, then distance along the perpendicular line from the great circle to the point being located (planet, star, etc.). The starting point for both Right Ascension and ecliptic longitude is the zero Aries intersection of the ecliptic and the equator.
In measuring the distance between two points on a sphere, measurements in any of the standard coordinate systems are usually a longer way around than the length of a great circle arc directly connecting the two points. (The great circle distance never exceeds 180 degrees.) Except when both points are exactly on the great circle of the coordinate system or on the same perpendicular to that great circle, the coordinate system measurement involves right angle turns from the great circle along the perpendiculars out to the points. Because of the angles involved, great circle separations (distances between two points such as two planets) are most similar to coordinate system separations around 90 degrees and most different around 0 and 180 degrees. Some people call the great circle arc directly connecting two points the true spherical distance.
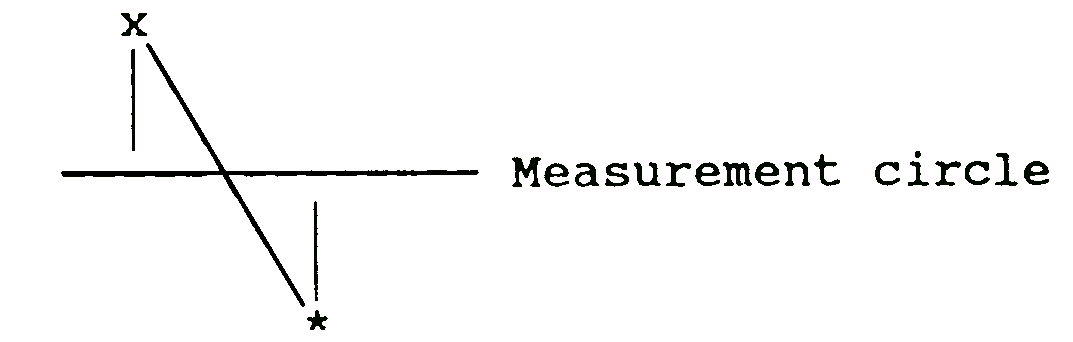
For those who can use it, here is a formula: Great circle distance = arccos(cos(latitude 1) * cos(latitude 2) * cos(longitude 1 - longitude 2) + sin(latitude 1) * sin(latitude 2)). (There is a fudge factor required for separations under 0.1 or over 179.9 degrees which I won't bother with here.) The longitudes and latitudes can be from any single coordinate system, but not from a mixture like zodiacal longitude with declination.
Aspects
The 1990 CCRS Horoscope Program allows you to list aspects or angular separations in geocentric longitude, heliocentric longitude, Right Ascension, azimuth, local azimuth, prime vertical longitude, galactic longitude, rationalized semi-arc, helio invariable plane, geo invariable plane, geo latitude, helio latitude, declination, altitude, local altitude, prime vertical amplitude, galactic latitude, helio invariable plane latitude, geo invariable plane latitude, or great circle arc. This gives considerable room to explore whether one system of measurement is better than another for aspects. While our visitor was here, I added a great circle arc option to the timed hits printout, so we can explore more and other people can try them when that goes out in the next release in a year or so (if I stick to my recent cycle).
Most of the time, we (and most astrologers) simply take the difference between the zodiacal longitudes of two planets as the total basis for judging whether they are in aspect and what aspect is involved. For most planets, this is a reasonable simplification. Except for Mercury and Pluto, most planets stay quite close to the ecliptic (along which we measure celestial/zodiacal longitude).
However, when we start using some asteroids (especially Pallas), we start running into noticeable celestial latitudes. Pallas can reach latitudes over 30 degrees, changing a zodiacal conjunction or opposition into a great circle semisextile or quincunx. If we start looking at fixed stars, we can be talking about very high celestial latitudes. Stars can be in great circle sextile or square to a planet they conjunct zodiacally. This kind of change makes ignoring latitude seem strange: calling two points conjunct when they are separated by one third or one half of the visible sky.
On the other hand, great circle distances used with reasonably tight orbs can completely lose a lot of conjunctions and oppositions, two aspects considered very important. (You lose conjunctions any time the latitude difference between your two points is greater than your orb. Oppositions are similar, except you tend to lose the ones where both points are north or both are south.) Maybe this could be used as an argument that the only important conjunctions are the ones where the latitudes are also close, just as most astrologers already treat eclipses as more important than other Sun-Moon conjunctions and oppositions.
Timing
In current patterns, besides the question of whether an aspect is present or not, we also have the question of when is it in orb and when is it exact (bringing back the 4th dimension we dropped earlier). Which aspects you see when depends on your choice of coordinate system, with each coordinate system (and great circle arcs) giving you either different aspects at the same time or the same aspects at different times.
Choices
Perhaps we need to open up our concepts of aspects. Maybe we can continue to use longitude aspects for most zodiacal work with most planets, use Right Ascension for diurnal work (in a sense, that is what Parans do), use azimuth or Prime Vertical longitude for local work, use galactic longitude for work with stars and use great circle distances for bodies with high latitudes or any mixture of systems. Has anyone out there done a study of various aspect measurements against events in people's lives or any other verifiable criterion? I consider the question of how to measure aspects to still be wide open.