Basic Astronomy
Mark Pottenger
Mathematics and science are suffering in our society from over-mystification. They are often presented in ways that make them seem more complicated and incomprehensible than they are. In this brief article, I am presenting very little more than definitions of terms. I think they are needed because too much is said and done without full grasp of meanings. (I tend to jump on connotations in conversation.) In future articles I will present math and astronomy at various levels—this is just a rather long introduction.
Any science (e.g., astronomy) is simply an attempt to understand the world or some facet of it. Mathematics is just another language (or family of languages) used, like any spoken language, to permit the representation of reality. Numbers (1, 2, 3, ...), operators (+, -, x, /) and functions (sine, log, ...) are just like nouns, verbs and modifiers in English, but better behaved. The math an astrologer needs nowadays is very little—all the hard work has been done in preparing the ephemerides and tables of houses. Ephemerides are books giving daily planetary positions. They are usually given for either zero hours (midnight) or 12 hours (noon) Universal Time or Ephemeris Time. Tables of houses give house cusps through the zodiac and over a range of latitudes. With the current availability of very low cost calculators, even what little math there is is made much easier.
The basic tool of the astrologer is the natal chart, or horoscope. The root parts of the word “horoscope” are “hour” and “view”, and that is what it is—a look at the world at a specific moment. If one thinks of it as a celestial snapshot, some things are clarified. A snapshot is taken at a particular time and place, looking in a particular direction. A horoscope is a picture of the sky at a specific moment, viewed from some point on Earth and looking toward that part of the sky where the planets can be seen. (Looking south for northern hemisphere observers north of the tropics.)
The planets always appear in a limited portion of the sky because of the geometry of the solar system. All planets revolve around the sun near a single plane (flat surface, sheet), with minor variations above and below. If you look at a plane from one side, holding it at eye level, what you see is a line. The ecliptic is the line formed by the plane of Earth’s orbit (path around the Sun). It can also be described as the apparent path of the Sun around the Earth. All planets appear within a few degrees (360ths of a circle) of the ecliptic.
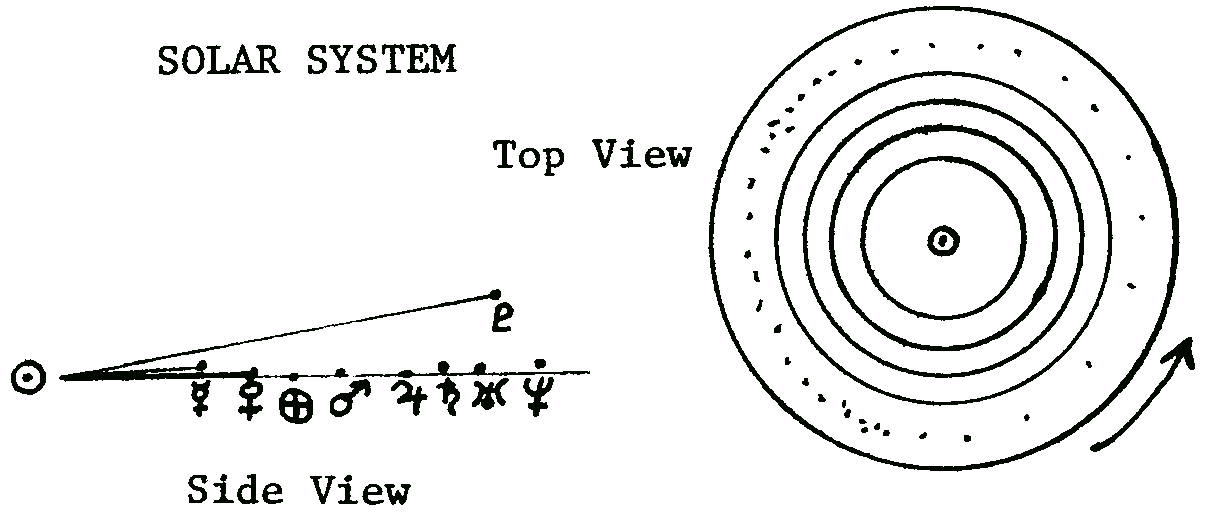
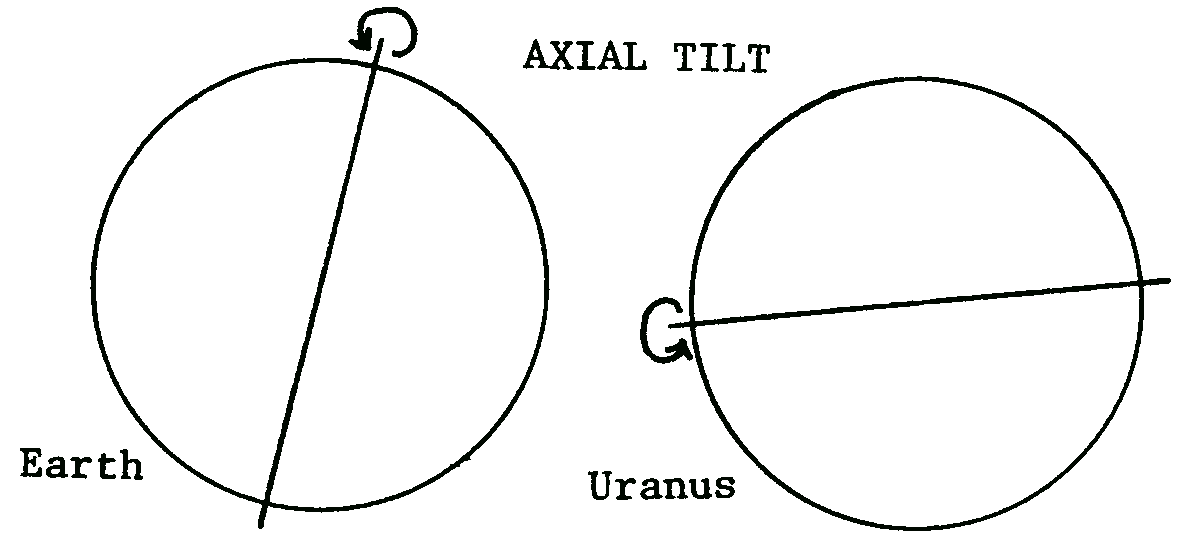
If you get out of the plane of the solar system and view it from above, the paths the planets follow appear nearly circular. They are actually very slightly elliptical (oval). When you are looking down from above the solar system, almost all motions appear counterclockwise. The planets revolve in their orbits counterclockwise and most planets rotate counterclockwise on their axes. (Any rotating object has an axis—the center line about which it rotates.) The only known exceptions are Venus, which rotates clockwise, and Uranus, which rolls along on its side (its axis is almost parallel to the plane of its orbit, instead of perpendicular like the rest of the planets). It would all look like a slowed down amusement park mad teacup—everything spinning and moving at once.
Returning to the horoscope as a celestial snapshot, the three basic elements of the chart—houses, planets and signs—can be compared to the foreground, middle and background. The houses are a division of the sky based on the Earth’s daily rotation. Your location on Earth, your time of day and your date in the year are all needed to get them. Being the foreground, the houses are the most rapidly changing part of the picture. The planets all move slowly enough, and are far enough from Earth, that they appear to be in the same place no matter where on Earth you view them from—so all you need are time and date. (Actually, their apparent positions do change from various viewpoints, but the change is usually small enough to ignore.) The signs are the background in terms of which the houses’ and planets’ positions are described.
Unlike a picture, the chart does not show the depth that is present. All information on distances and deviations from the plane of the ecliptic are squeezed out of the chart so it can be drawn on a flat circle. This circle is the ecliptic, and the planets are shown on it even though they are sometimes a few degrees away. The house cusps are also just a part of the houses, since they extend all the way around the celestial sphere. Eliminating visual information from a picture and adding labels turns it into a kind of map—and that is what the horoscope is—a sky map (look up “chart” in a dictionary). Since the ecliptic is south of most people in the northern hemisphere, the chart is like a map looking south—south is at the top (Midheaven) and east is at the left (Ascendant).
Coordinate Systems
The Earth is a sphere (actually, not quite—but the deviation is small enough to ignore most of the time). Once a frame of reference is established, the location of any point on a sphere can be specified with just two coordinates. (A coordinate is a number that says “go this distance in this direction”.) On Earth, those two coordinates are latitude and longitude.
A reference point to measure from is needed in any coordinate system. For a spinning sphere like the Earth one reference is automatic. The poles (where the axis of rotation touches the surface of the sphere) can be found by observation. Measurement north and south between the poles is latitude. The equator is the great circle perpendicular (at 90°) to the axis or rotation, or exactly halfway between the poles. (A great circle is a circle made by a plane that goes through the center of a sphere. It is the largest circle possible on that sphere. If you cut an orange exactly through the middle, the peel you see is a great circle. If you cut away from the center, the peel shows a lesser circle.) On Earth, latitude is defined to be zero at the equator, going up and down 90° to the two poles.
The other coordinate on Earth is longitude. It is measured east and west around the equator. However, there is nothing obvious like the poles to start counting from, so an arbitrary starting point is necessary. When the terrestrial coordinate system was finalized last century, the zero point chosen was a specific instrument in the observatory at Greenwich, England. Longitude goes 180° east and west from Greenwich. A line of equal longitude (a line going north-south) is called a meridian. The line of longitude passing through Greenwich is called the Prime Meridian.
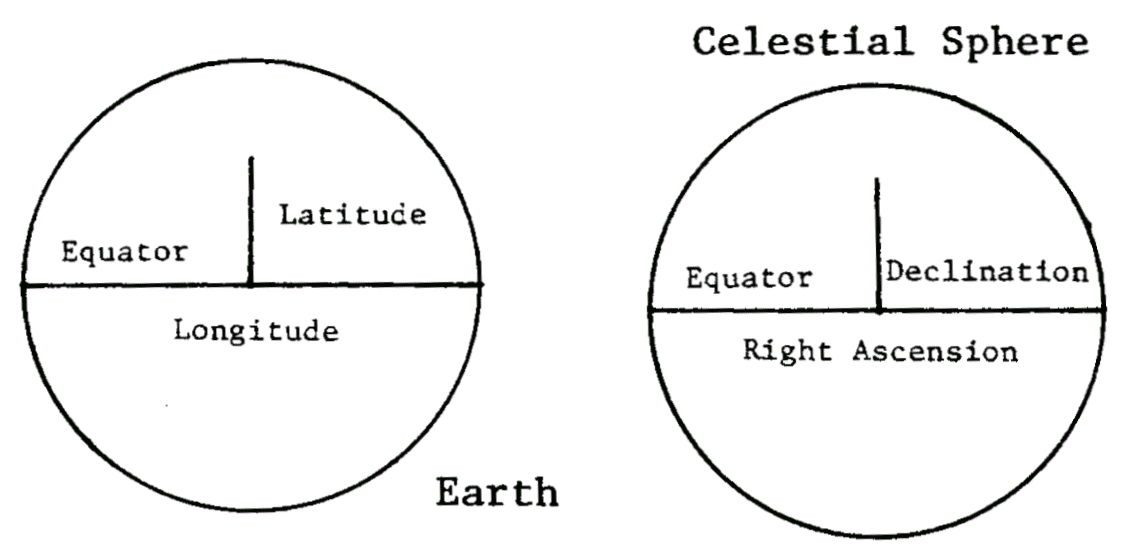
Celestial coordinates follow the same principles with only a few differences in detail. The “celestial sphere” is a convenient fiction used to permit easy description of coordinates. Everything in the sky is assumed to be the same distance away, on the surface of a sphere, rather than at the varying distances that actually exist. All motion is assumed to be in the sky—Earth is assumed to be the stationary center of everything. The celestial sphere is said to rotate, although that is just the visual effect created by Earth’s rotation.
This apparent rotation gives the celestial sphere an equator and poles to match Earth’s (in fact, they are outward extensions or projections of Earth’s equator and poles). Most astronomical coordinates are given in terms of declination (Dec) north and south of the celestial equator and Right Ascension (RA) along the celestial equator.
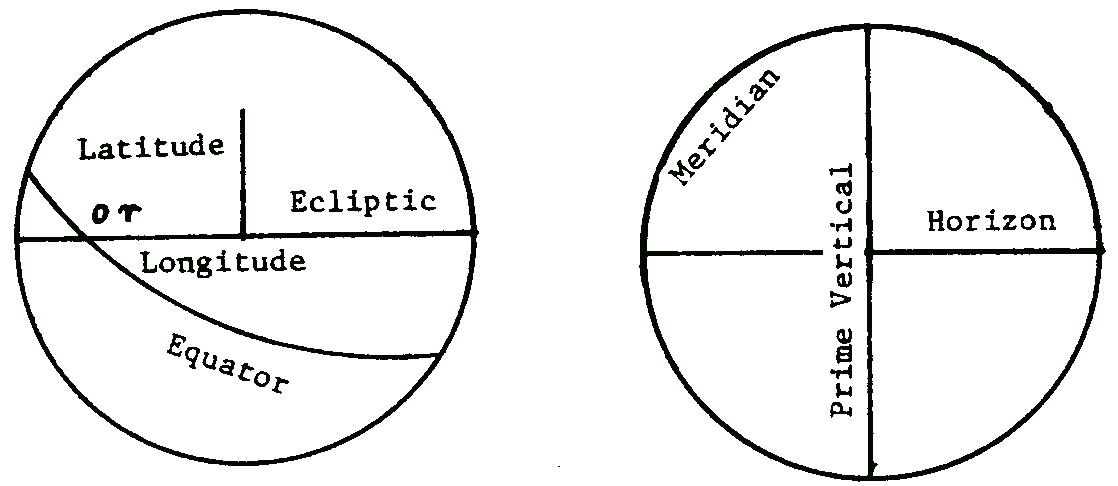
In the sky there are features that establish a number of important great circles in addition to the equator. The ecliptic, or Earth-Sun path, is a great circle on the celestial sphere. The coordinate most used by astrologers is celestial longitude measured around the ecliptic. Celestial latitude measures north and south from the ecliptic to the poles of the ecliptic. (Any great circle has poles. Run a straight line through the center of the sphere, perpendicular to the great circle—where the line intersects the surface of the sphere you have poles.)
There are also coordinates going along, and north and south from, the plane of the milky way galaxy in which we live. Another set of coordinates important to astrologers is based on the observer’s horizon—altitude is measured up and down from it, and azimuth along it (around it). The three great circles from this system—the (rational) horizon, the observer’s meridian and the prime vertical—are important in determining house cusps and angles. The rational horizon is the great circle passing through the center of the Earth and parallel to the actual horizon—perpendicular to the line from zenith to nadir. The observer’s meridian is the great circle passing through the zenith and the north and south points of the horizon. The prime vertical is the great circle passing through the zenith and the east and west points or the horizon. The zenith is the point straight overhead for the observer, and the nadir is opposite this straight underfoot.
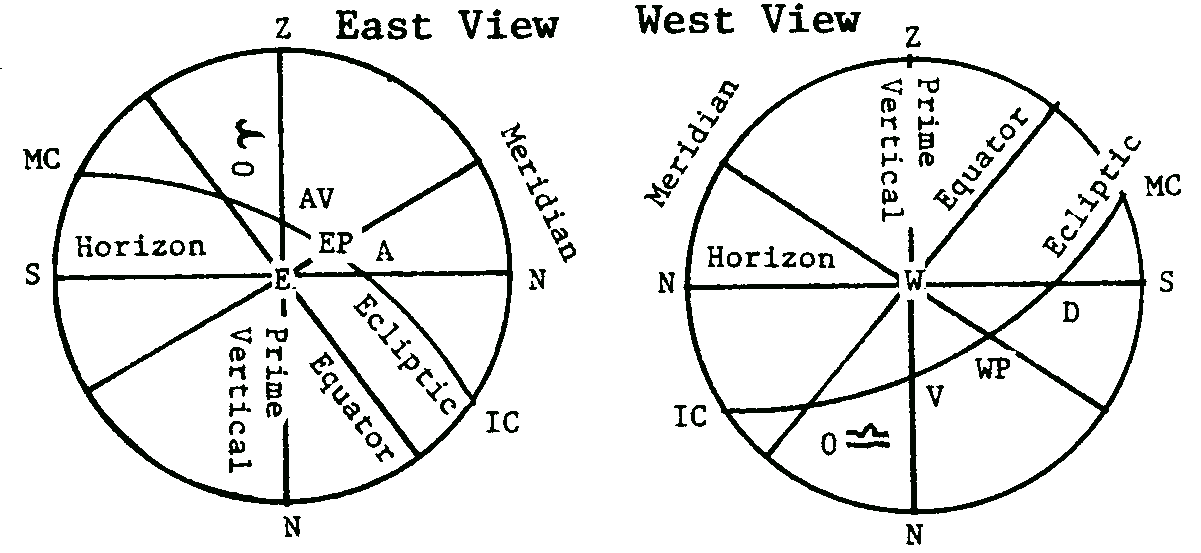
Any one of these coordinate systems can be used, or more than one at a time. It is even possible to use one coordinate from one system and one from another and still describe a point precisely, but it is cumbersome. Astrologers actually do this by using longitude from the ecliptic system and declination from the equatorial system. Declination is used because the planets vary much more in declination than in latitude. Interactions between the horizon, ecliptic and equatorial coordinate systems provide the angles and houses.
Any pair of great circles will intersect at two opposite points—try stretching rubber bands around a ball to get some idea of circles on a sphere. The intersection of the ecliptic and equator provides something missing on Earth—a natural starting point to measure from. The Earth’s zero longitude or Prime Meridian was set arbitrarily. The zero point for both Right Ascension and celestial longitude is the zero Aries intersection of ecliptic and equator. This is the vernal equinox point—where the Sun is at the official start of northern hemisphere spring. Celestial longitude is measured east around the ecliptic in a full 360 degrees—not 180 degrees each way like on Earth. Every 30 degrees along the ecliptic is a tropical astrological sign, starting with zero Aries at the vernal equinox point.
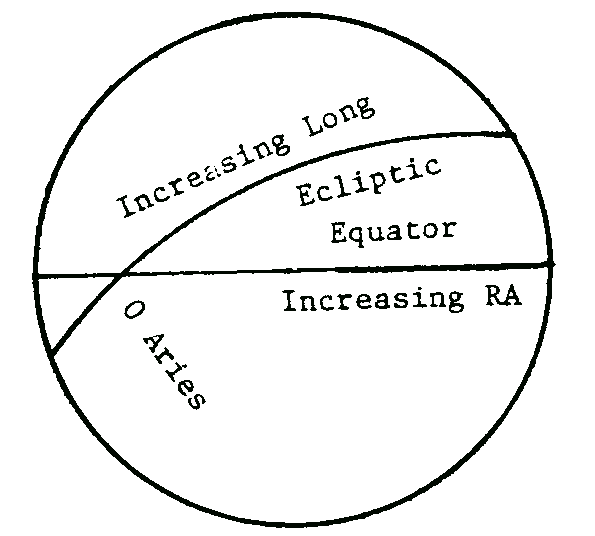
Right Ascension is also measured full circle east from the vernal equinox rather than half and half, but it is not usually measured in degrees. RA divides the equator into hours, minutes and seconds (hms). It is possible to measure RA in degrees, minutes and seconds (dms), but if you convert from one to the other remember that the minutes and seconds in the two systems are different units. With dms you divide the circle by 360, then 60, then 60 again. With hms you divide the circle by 24, then 60, then 60. Thus, we have a factor of 15 difference which it is not safe to confuse.
360 degree circle 24 hour circle (or day)
Units:
Degrees 1/360 hours 1/24
Minutes 1/60 ° Minutes 1/60 h
Seconds 1/60 ’ Seconds 1/60 m
360° = 24 hours
30° (1 sign) = 2 hours
15° = 1 hour
1° = 4 minutes
15’ = 1 minute
1’ = 4 seconds
15” = 1 second
1” = 1/15 second
Times
Most units of time have some astronomical basis, or are arithmetic manipulations of such units. The day is the length of time it takes the Earth to make one complete rotation on its axis. The hour (hr, h) is one twenty-fourth of a day. The minute (min, m) is one sixtieth of an hour. The second (sec, s) is one sixtieth of a minute. A millisecond is one thousandth, and a microsecond one millionth of a second. Going to larger units, a week is one quarter of a lunar cycle—the time from one obvious phase to the next (new, quarter or full). A month was originally the time it took the moon to make one complete revolution around the Earth—now it is a day or two longer than that. A year is the time is takes the Earth to make one complete revolution around the Sun. A century is a hundred years, a millennium a thousand, and an aeon a billion.
In setting up charts, the astrologer deals with a somewhat confusing variety of times. Most of the units just defined vary depending on what kind of reference you use. Sidereal time is time determined by Earth’s rotation with respect to the stars. Solar time is determined by Earth’s rotation with respect to the Sun. Because the Sun is tremendously closer to us than even the nearest stars (millions vs. trillions of kilometers), a slight change in the Earth’s position causes the Sun to appear to move without causing the stars to appear to move. This phenomenon of near objects seeming to move more than far ones is called parallax and is used in astronomical measurements. Back to our snapshot analogy, if you move the camera a little the foreground changes much more than the background. Compared to the stars, the whole solar system is foreground.
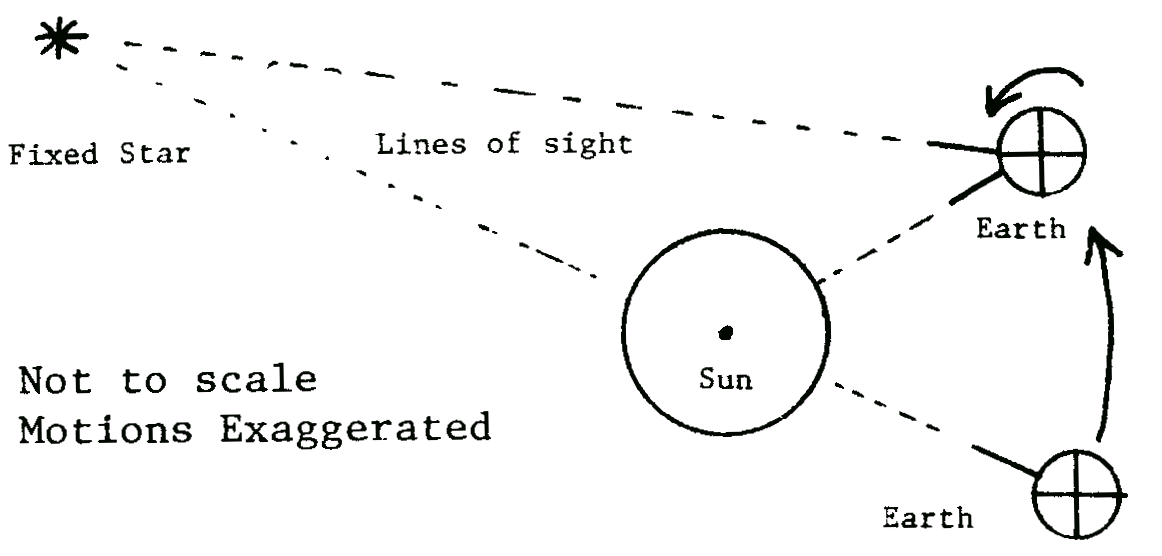
Since the Earth moves about one degree in its orbit in a single day, by the time it completes one rotation with respect to some fixed star the Sun is a degree away from a complete rotation. Looking at the diagram, which shows Earth’s rotation and revolution for one day (both counterclockwise since this is a top view), we see that the degree still has to be traveled to complete the rotation with respect to the Sun. Thus, a solar day is longer than a sidereal day.
Mean (average) time is any time that has had irregularities averaged out. Almost all celestial (and terrestrial) motions contain some unevenness, so mean time is necessary to match the evenness of manufactured clocks. A sundial, which shows true (not mean) sun time, can differ from clocks by as much as seventeen minutes during part of the year. You can have both mean solar and mean sidereal times. One mean sidereal day is 23h 56m 4.09054s of mean solar time. Conversely, one mean solar day is 24h 3m 56.55536s of mean sidereal time. Because of this difference, solar and sidereal time agree only once a year—near the autumnal equinox. Each day after that, sidereal time gets later than solar time by four more minutes, making a complete cycle in a year.
Local Mean Time, also called True Local Time or Sun Time, (LMT, TLT) is the mean solar time for any particular longitude. Local Sidereal Time (LST) is the mean sidereal time for a particular longitude. Greenwich Mean Time or Universal Time (GMT, UT)—also sometimes referred to as Greenwich Civil Time—is the mean solar time at the Prime Meridian (0° longitude) running through Greenwich observatory. The mean sidereal time there is called Greenwich Sidereal Time. The Sidereal time given at the start of each day’s entries in an ephemeris is the Greenwich Sidereal Time at midnight (or noon, if you use a noon ephemeris). Ephemeris Time (ET), used in most modern ephemerides, is only a few seconds different from Universal Time. ET is more uniform than any time based on the Earth’s daily rotation (which is not steady)—it is based on the motions of the whole solar system.
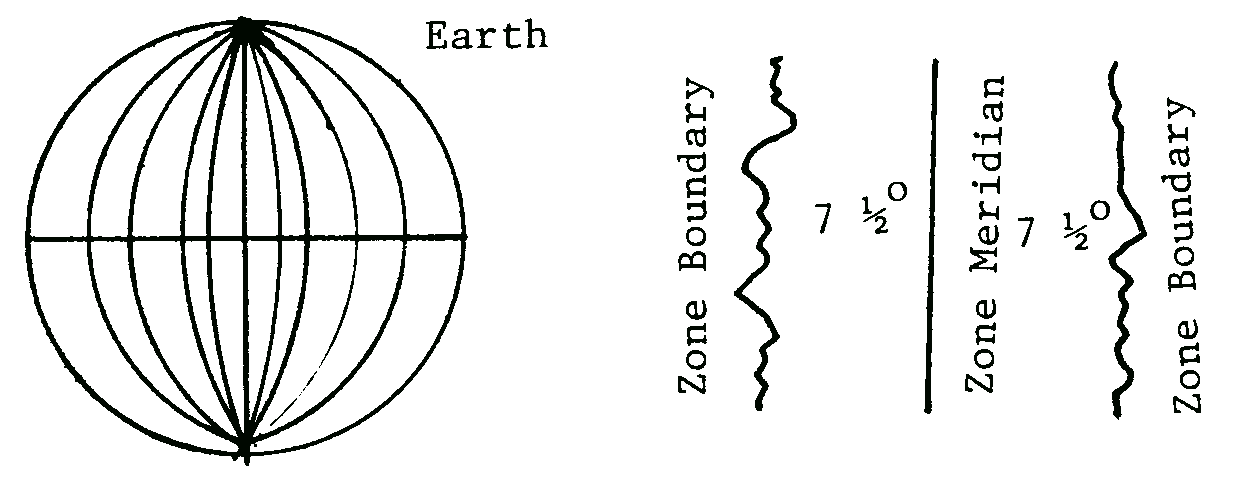
The Earth has been divided into 24 one hour wide standard time zones. As we already saw with Right Ascension, one hour equals fifteen degrees when we match 24 hours to 360 degrees. Thus, each zone is 15° of longitude wide. Standard time started less than a hundred years ago when increasingly rapid communication made it desirable to have the same time over a large area. Before it was adopted any change of longitude meant a change of time, and scheduling was confusing. With Standard time, a whole 15° (1 hour) wide zone adopts the local mean time of the central meridian for that zone. The boundaries are approximately 7 1/2° from the central meridian, but wander a lot in attempts to avoid populous areas. For places at the western edge of a zone, standard time is about a half hour later than local mean time. For places at the eastern zone edge, standard is about a half hour earlier than local mean time. Since the zones are one hour wide, zone times differ by some whole number of hours (there are only a few half and quarter hour zones in the world).
The Prime (Greenwich) Meridian is the central line for a time zone. Since zone meridians are 15° apart and Greenwich is 0° longitude, every even hour zone meridian is at some whole multiple of 15 degrees of longitude. In the U.S., 60° west longitude is the meridian for zone 4 or Atlantic [Standard] Time (A[S]T), 75° W is zone 5 or Eastern Time (ET), 90° W is zone 6 or Central Time (CT), 105° W is zone 7 or Mountain Time (MT), 120° W is zone 8 or Pacific Time (PT), 135° W is zone 9 or Yukon Time (YT), 150° W is zone 10 or Alaska-Hawaii Time (AHT), and 165° W is zone 11 or Bering Time (BT). Because of the 1:15 ratio between hours and degrees, dividing the longitude by 15 gives the zone number and multiplying the zone number by 15 gives the longitude of the zone meridian. The zone number gives the number of hours difference between Standard time for that zone and Universal Time.
Daylight (or Summer or War) Time is Standard time plus one hour, or, restated, the standard time of the next zone to the east. Double Summer (or double daylight) time is standard time plus two hours, or the standard time of a zone two to the east. It doesn’t save daylight—it saves power. It shifts the hours of daylight later in the day so lights don’t have to be turned on as early (and there is light for more late afternoon leisure activity). It shifts darkness later into the morning, but not to a time when many people are up.
A note on abbreviations. If a time is given as PST, PDT or PWT, that is to be read as Pacific Standard Time, Pacific Daylight Time or Pacific War Time. The first letter (or sometimes two as in Alaska-Hawaii) gives the zone and the second says what type of time was being observed. Slightly confusing to Americans is the abbreviation BST—this is British Summer Time.
The Solar-Sidereal Correction (or Acceleration Interval) is a correction to change a given interval of mean solar time into an equivalent interval of mean sidereal time. This is 3 minutes 56 1/2 seconds per day or 9.856 seconds per hour or about 1 second every 6 minutes added to a solar time interval to get an equivalent sidereal time interval. (Interval is time between two instants.) Thus, Greenwich Mean Time (Universal Time) Interval is the time between the midnight or noon for which an ephemeris is set and the Greenwich Mean Time (UT) of birth (or whatever). (With a midnight ephemeris GMTI (UTI) = GMT (UT) expressed in 24 hour time. For example if UT of birth = 10:23 then UTI for Midnight ephemeris = 10:23-0 = 10:23.) Adjusted True Local Time is the sidereal time interval since local midnight, obtained from True Local Time by using the Solar-Sidereal Correction (Acceleration Interval).
A Longitude-Time Correction is the conversion of an arc of longitude (360° circle) to an equivalent time interval (24 hour circle). We already discussed this when talking about Right Ascension. I repeat the warning about mixing up the two types of minutes and seconds. A Longitude Time Correction can be applied to either a solar or a sidereal time.
The important thing to remember in the face of all these types of time is that whatever time you are working with, all you are doing is expressing it in a different way. For example, 1100 EST = 1200 EDT = 1600 GMT = GMTI of 1600 for midnight ephemeris = 11:04:12 LMT in New York City, etc. A very helpful point to remember is that east is always later and west is always earlier—both here on Earth and in the visible (above the horizon) sky.
Having subjected you to all that, I am open to suggestions from readers as to what level and subject matter (math or astronomy) they would like next issue. If this was too basic, not basic enough, or just about right, say so.